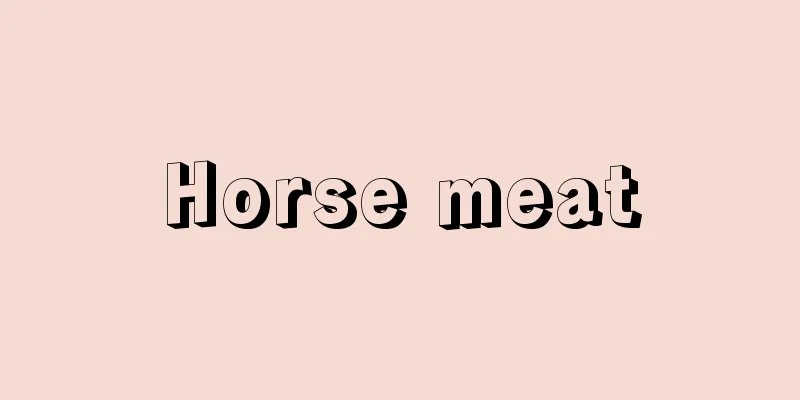Poisson distribution

|
With λ>0 The Poisson distribution P(λ) can be expressed as a line graph as follows. If λ is an integer, p k increases monotonically when k < λ-1, reaches a maximum value when k = λ-1, k = λ, and decreases monotonically when k > λ. If λ is not an integer, p k is k < [λ] = (the integer part of λ) [Shigeru Furuya] Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend |
|
λ>0として ポアソン分布P(λ)を折れ線グラフで表すと次のようになる。λが整数であれば、pkはk<λ-1のとき単調増加で、k=λ-1,k=λのとき最大値をとり、k>λで単調減少である。λが整数でなければpkは [古屋 茂] 出典 小学館 日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について 情報 | 凡例 |
<<: Poitiers (English spelling)
Recommend
The puppet's grandson
...This book lists the famous places where they g...
Davis Strait
A strait between the eastern end of Baffin Island...
Gabbro - Gaburo
Please see the "Gabbros" page. Source: ...
Vésone (English spelling)
...Population: 33,000 (1990). Originating from th...
chirurgy
…In Japan, the word “surgery” is used because it ...
Souma Kokkou
Essayist. Born into a samurai family in Miyagi Pr...
Red-breasted Lapwing - Red-breasted Lapwing
...The Peruvian lacewing Phytotoma raimondi is fo...
Mount Kariba
It is the main peak of the Kariba Mountains at th...
Imatomi-myo
...The center of the area is currently Obama City...
Kawagoe Railway - Kawagoe Railway
...The main lines are the Ikebukuro Line between ...
Priapulus bicaudatus
… [Minoru Imajima]. … *Some of the terminology th...
Tenor - Tenor (English spelling)
A musical term. Also called tenor, it has the fol...
Supreme Court - saikō saibansho
The highest organ of the state responsible for ju...
Agitator dryer - Agitator dryer
...A type in which the material moves on a band i...
Chinju no Mori - Shrine Forest
The grove surrounding a shrine dedicated to the gu...