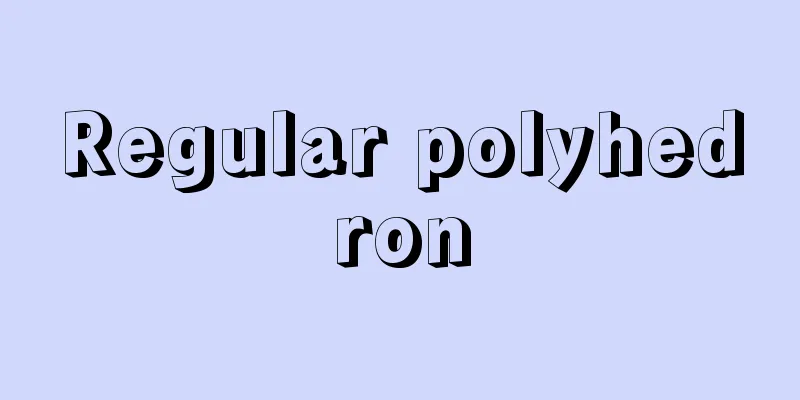
Regular polyhedron

Japanese: 正多面体 - せいためんたい（英語表記）regular polyhedron

A polyhedron in which all faces are made of congruent regular polygons and the angles around all vertices are equal. There are only five types of regular convex polyhedrons: the tetrahedron, the cubic octahedron, the dodecahedron, and the icosahedron. These are sometimes called Platonic solids. The number of vertices v , the number of edges e , and the number of faces f of these regular convex polyhedrons are related by the equation v - e + f = 2 (→ Euler's theorem). If we consider the movement of superimposing each of the five regular polyhedrons above on itself, and consider this as a permutation between the vertices, we can see that this movement forms a finite group. The orders of the finite groups formed by superimposing the tetrahedron, hexahedron, octahedron, dodecahedron, and icosahedron on themselves are 12, 24, 24, 60, and 60, respectively, depending on the product of the number of vertices v and the number of faces that meet at the vertices g . This finite group of regular polyhedrons is called the group of regular polyhedrons. For example, a regular tetrahedron is called a regular tetrahedron group. There are four types of star-shaped regular polyhedrons: Kepler-Poinsot.

Source: Encyclopaedia Britannica Concise Encyclopedia About Encyclopaedia Britannica Concise Encyclopedia Information

Japanese:

すべての面が互いに合同な正多角形から成り，しかもすべての頂点の周りの面角が等しい多面体をいう。凸正多面体には，正四面体，正六面体，正八面体，正十二面体，正二十面体の5種類しかない。これらをプラトンの立体ということがある。これら凸正多面体の頂点の数 v ，辺の数 e ，面の数 f の間には v－e＋f＝2 という関係がある (→オイラーの定理 ) 。また上の5つの正多面体について，それぞれ自分自身の上に重ね合せる運動を考えると，これを頂点間の置換とみなせば，この運動は有限群をつくることがわかる。正四，六，八，十二，二十面体を，それぞれ自分自身の上へ重ね合せる運動がつくる有限群の位数は，頂点の数 v と頂点に集まる面の数 g の積によって，それぞれ 12，24，24，60，60となる。正多面体についてのこの有限群のことを正多面体群という。たとえば正四面体については正四面体群などという。なお星形正多面体は，ケプラー＝ポアンソの4種類がある。

出典　ブリタニカ国際大百科事典小項目事典ブリタニカ国際大百科事典小項目事典について　情報

<<: Seidan [town] - Seidan

>>: Danso Kiyota