Extreme value

Japanese: 極値 - きょくち（英語表記）optimum value

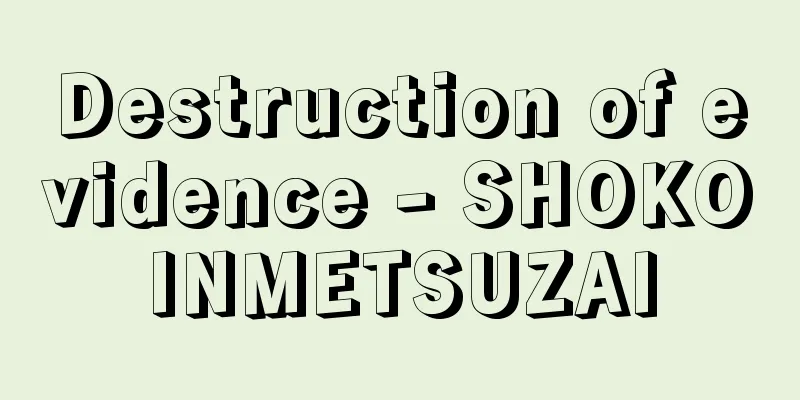
When a function is given, if we take a point P and take a point V in its vicinity, and if the function is maximum (minimum) at P in V, then the function is said to be maximum (minimum) at P, and the function value at that time is called the maximum (minimum). Maximum and minimum values are collectively called extreme values.

Since ancient times, an important problem in mathematics has been to find the points where a function has a maximum or minimum within the entire domain of the function. However, since the properties of maximum and minimum are global, it is generally very difficult to find a solution. On the other hand, the properties of maximum and minimum are local, so points that give extreme values can be found using differentiation, as shown below. A practical approach is to find the points that give the maximum and minimum from among the points that give these extreme values.

[Osamu Takenouchi]

Extrema of a function of one variable

If a function f(x) is differentiable at x = x ₀ and has an extremum at x = x ₀ , then f'(x ₀ ) = 0. If f'(x) is positive to the left of x ₀ and negative to the right, then x ₀ gives a maximum of f(x), and if it is negative to the left and positive to the right, then x 0 gives a minimum. Also, if f(x) is differentiable n times at x = x ₀ , then
f′(x ₀ )=0, f″(x ₀ )=0,……,
f ^(n-1) (x ₀ )=0, f ⁽ⁿ⁾ (x ₀ )≠0
When n is even, x ₀ is a point that gives an extreme value of f(x), and when n is odd, x ₀ does not give an extreme value of f(x).

[Osamu Takenouchi]

Extrema of a function of several variables

To simplify the description, we will discuss the case of a function of two variables. If f(x, y) is partially differentiable with respect to P(x ₀ , y ₀ ) and f takes an extreme value at P, then f _x (x ₀ , y ₀ )＝0, f _y (x ₀ , y ₀ )＝0 (f _x and f _y are the partial differential coefficients of f). Also, suppose f is twice continuously differentiable in the vicinity of P (f _xx , f _xy , and f _yy exist and are continuous), and f _x (x ₀ , y ₀ )＝0, f _y (x ₀ , y ₀ )＝0.

Δ = f _xy ( x ₀ , y ₀ ) ²
-f _xx (x ₀ , y ₀ )f _yy (x ₀ , y ₀ )
Depending on the sign of the value, if Δ < 0, f is determined to be an extreme value at P, and if Δ > 0, it is determined to be a non-extreme value. However, when Δ = 0, it is not possible to determine either from this alone.

When x and y change under the condition ∅(x, y)＝0, the points with these values form a curve. To find the maximum and minimum of the function f(x, y) on this curve, consider a constant λ and create the equation f(x, y)－λ∅(x, y), and set the partial derivative of this with respect to x and y to zero, that is,
f _x (x, y)－λ∅ _x (x, y)＝0,
f _y (x, y)－λ∅ _y (x, y)=0
We can then examine the points (x, y) that satisfy the following formula as candidates for maximum and minimum. This method is called the Lagrange method of undetermined multipliers.

[Osamu Takenouchi]

How to find extreme values of functions of multiple variables
©Shogakukan ">

How to find extreme values of functions of multiple variables

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

関数が与えられたとき、1点Pの適当な近傍Vをとると、Vのなかではその関数がPにおいて最大（最小）となっているとき、関数はPにおいて極大（極小）となるといい、そのときの関数値を極大値（極小値）という。極大値、極小値を総称して極値という。

　関数が最大あるいは最小になる点が存在するならば、それを関数の全定義域から求めよ、というのは、古来数学における重要な問題であった。しかし、最大・最小という性質が大域的なものであるため、一般的に解を得ることはたいへん困難である。一方、極大・極小という性質は局所的なものであって、極値を与える点は、以下に示すように微分法を用いて探すことができる。これら極値を与える点から最大・最小を与えるものを求める、というのが実用的なやり方である。

［竹之内脩］

一変数関数の極値

関数f(x)がx＝x₀で微分可能でx＝x₀で極値をとるならば、f′(x₀)＝0である。もしf′(x)がx₀の左側では正、右側では負であれば、x₀はf(x)の極大を与え、左側で負、右側で正ならば極小を与える。またf(x)がx＝x₀でn回微分可能で、
　f′(x₀)＝0, f″(x₀)＝0,……,
　f^(n-1)(x₀)＝0, f⁽ⁿ⁾(x₀)≠0
のときは、nが偶数ならばx₀はf(x)の極値を与える点であり、nが奇数のときはx₀はf(x)の極値を与えない。

［竹之内脩］

多変数関数の極値

記述を簡単にするため二変数関数の場合を述べる。f(x, y)がP(x₀, y₀)で偏微分可能で、fがPで極値をとるならば、f_x(x₀, y₀)＝0, f_y(x₀, y₀)＝0である（f_x、f_yはfの偏微分係数）。また、fがPの近傍において2回連続微分可能（f_xx、f_xy、f_yyが存在して連続）で、f_x(x₀, y₀)＝0, f_y(x₀, y₀)＝0であるとする。

　Δ＝f_xy(x₀, y₀)²
　－f_xx(x₀, y₀)f_yy(x₀, y₀)
なる値の符号によって、Δ＜0ならばfはPで極値をとり、Δ＞0ならば極値をとらないと判定される。ただしΔ＝0であるときは、これだけからはいずれとも判定できない。

　∅(x, y)＝0という条件のもとでx、yが変化するとき、これらの値をもつ点は一つの曲線を描く。この曲線上での関数f(x, y)の極大・極小を求めるには、一つの定数λを考えて、f(x, y)－λ∅(x, y)という式をつくり、これをx、yに関して偏微分したものをゼロと置いて、すなわち、
　f_x(x, y)－λ∅_x(x, y)＝0,
　f_y(x, y)－λ∅_y(x, y)＝0
を満たす点(x, y)を極大・極小を与える候補として調べればよい。この方法をラグランジュの未定乗数法という。

［竹之内脩］

多変数関数の極値の求め方

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例

<<: Polar Urals

>>: Polar Regions