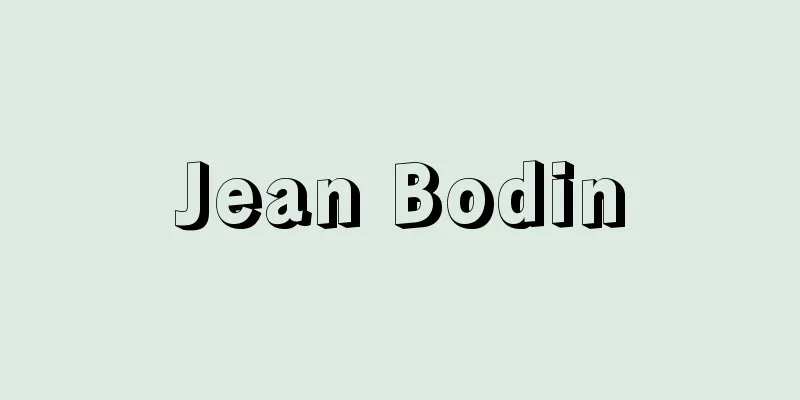
Square root

When a number a is given, the number x that, when squared, gives a , in other words, the number x such that x ² = a, is called the square root of a . When a is a positive number, there is one positive and one negative square root of a , and their absolute values ​​are equal. The positive one is written as . is read as root a . The negative one is represented as -. Therefore, in this case, the square root of a can be summarized as ±. For example, the square root of 2 is and -, which can be summarized as ±. In this case, the symbol is called a radical sign (or square root sign). The square root of 0 is just 0. Also, the square root of a negative number is not a real number, but an imaginary number. For example, the square root of -2 is i and -i ( i is the imaginary unit).

Let's consider the square root of a positive integer. When a is a square number, that is, a number that can be written as the square of a positive integer n , n ² , then a is equal to n (for example, a = 2). However, when a is not a square number, a is never a rational number, but an irrational number. In other words, it can never be expressed as a finite decimal or fraction, but rather as a non-repeating infinite decimal (for example, a = 1.41421356...). If the length of one side of a square is 1, then the length of the diagonal can be expressed as, but the ancient Greeks already knew that a was an irrational number. This can be seen in Euclid's Elements.

When we are considering the square root of a positive number, the radical symbol always represents a positive number. This is the convention for the radical symbol. So, when a > 0, it is = a , but when a < 0, a ² > 0, so it is = - a .

Sometimes the square root is contained within the square root sign. For example,

In such cases, it is generally not possible to remove the double square root sign, but there are cases where it is possible. When a and b are positive integers and is an irrational number,

Positive rational numbers x and y such that exist only if a ² - b is a square of a rational number. For example,

So, 2 ² - 3 = 1 = 1 ² ,

However,

The square root sign cannot be removed.

[Tatsuro Miwa]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

数aが与えられたとき、二乗（平方）してaとなる数、つまり、x²＝aとなる数xをaの平方根という。aが正の数のときは、aの平方根は正の数、負の数それぞれ一つずつあり、その絶対値は等しい。そして、正のほうを、と書く。は、ルートaと読む。負のほうは、－で表される。したがって、このとき、aの平方根は、±とまとめられることになる。たとえば、2の平方根は、と－で、±とまとめられる。この場合、記号を、根号（または平方根号）という。0の平方根は0だけである。また、負の数の平方根は、実数でなく、虚数である。たとえば－2の平方根は、iと－iである（iは虚数単位で、のこと）。

　正の整数の平方根について考える。aが平方数、つまり、ある正の整数nの平方の形n²と書かれる数であるときは、はnに等しい（たとえば　＝2）。しかし、aが平方数でないとき、は有理数になることはけっしてなく、無理数になる。つまり、有限小数や分数で表されることはなく、循環しない無限小数になる（たとえば＝1.41421356……）。正方形の一辺の長さを1とすれば、対角線の長さはと表されるが、が無理数であることを古代ギリシア人はすでに知っていた。それは、ユークリッドの『原論』にみられる。

　正の数の平方根について考えているとき、根号はつねに正の数を表す。これが根号の規約である。すると、a＞0のとき＝aであるが、a＜0のときa²＞0であって、＝－aとなる。

　平方根号の中に平方根が含まれることがある。たとえば、

である。このような場合、一般には、二重の平方根号を外すことはできないが、外すことのできる場合がある。a、bが正の整数、が無理数のとき、

となる正の有理数x、yは、a²－bが有理数の平方の形になるときにだけ存在する。たとえば、

では、2²－3＝1＝1²で、

となる。しかし、

の平方根号は、外すことができない。

［三輪辰郎］

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例