Geometric mean

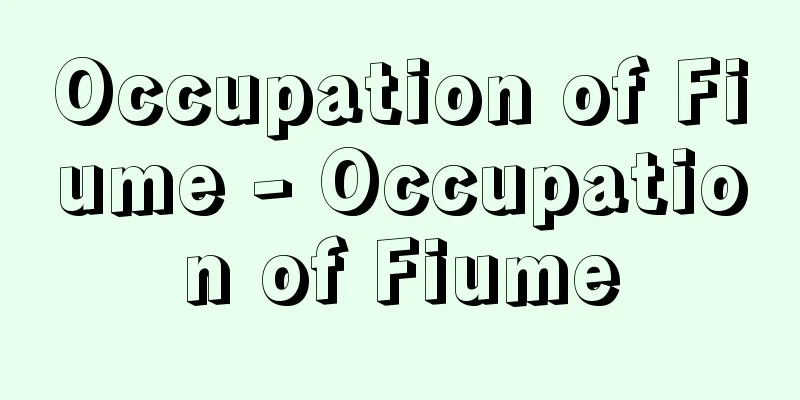
For n positive numbers a ₁ , a ₂ ,……, a _n , the nth root of the product of these n numbers

is called the geometric mean of a ₁ , a ₂ , …, a _n . It should be noted that the geometric mean is defined only when a _i (i=1,2,……,n) are all positive numbers. The geometric mean is used as a representative value for indexes such as price indexes and income distributions.

When _a1 , _a2 , ..., _an are positive numbers, let A, G, and H denote the arithmetic mean, geometric mean, and harmonic mean, respectively, and then H≦G≦A.
Here, if H=G, then _a1 = _a2 =……=a _n , and if G=A, then _a1 = _a2 =……=a _n . Also, if the geometric mean of positive numbers _ai (1≦i≦n) is G, then logG is the arithmetic mean of loga _i .

[Shigeru Furuya]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

n個の正数a₁,a₂,……,a_nに対して、これらn個の数の積のn乗根

をa₁,a₂,……,a_nの相乗平均または幾何平均という。相乗平均はa_i(i=1,2,……,n)がすべて正数のときに限って定義されるものであることに注意しなければならない。相乗平均は物価指数などの指数や所得分布の代表値として用いられる。

　a₁,a₂,……,a_nが正数のとき、これらの相加平均、相乗平均、調和平均をそれぞれA、G、Hで表すと
　　H≦G≦A
が成り立つ。ここでH=Gであればa₁=a₂=……=a_nであり、G=Aであればa₁=a₂=……=a_nである。また、正数a_i(1≦i≦n)の相乗平均をGとするとlogGはloga_iの相加平均になっている。

［古屋　茂］

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例