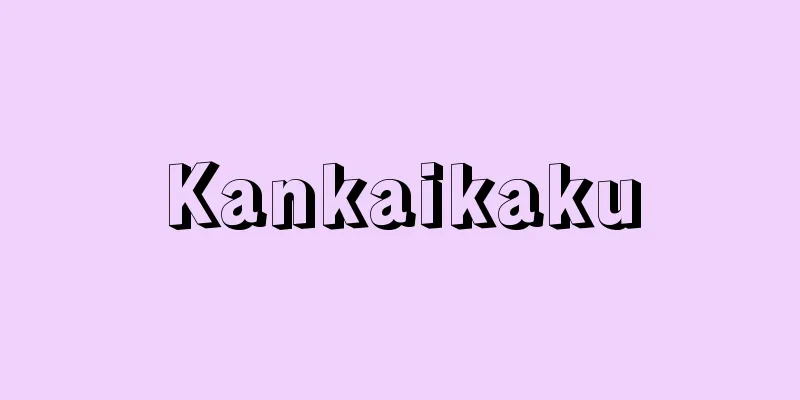Curve - Kyokusen (English spelling) curve

Japanese: 曲線 - きょくせん（英語表記）curve

A curve or continuous curve is a point on a plane or in space whose coordinates are a continuous function of a real variable t. A curve in a plane is called a plane curve, and the equation
x＝f(t), y＝g(t)
A curve in space is called a space curve.
x = f(t), y = g(t), z = h(t)
It is expressed as:

The curve is also
F(x, y)＝0
or
F(x, y, z)=0, G(x, y, z)=0
For example, a circle is given as
x＝ r cos t , y＝ r sin t
or
^x2 + ^y2 = ^r2
is given in the form:

A plane curve may also be given by y = u(x) or in polar coordinates by r = v(θ). In what follows, we will mainly consider plane curves.

For information on individual curves such as "asteroid," "cardioid," "clothoid," "conchoid," "cycloid," "sprint line," "tractrix," "spiral," and "limacon," please refer to the respective entries.

[Osamu Takenouchi]

Smooth curves

In the plane curve x = f(t) and y = g(t), when f(t) and g(t) have derivatives and are continuous, the curve is called a smooth curve. The vector (f'(t), g'(t)) with f'(t) and g'(t) as components is called the tangent vector. A smooth curve is a curve whose tangent vector changes continuously. A tangent vector is a vector that is connected between two nearby points on a curve.
P(t)＝(f(t), g(t))
P(t+ Δ t)=(f(t+ Δ t), g(t+ Δ t))
This is the limit obtained by dividing the vector connecting by Δt .

[Osamu Takenouchi]

Curve Length

When there is a curve connecting two points A and B, there are many points on the curve between A and B in order.
P ₁ , P ₂ ,……, P _n-1 (A=P ₀ , B=P _n )
These points are then connected in turn with line segments to create a broken line. If the length of this broken line (the sum of the lengths of each line segment) converges to a certain limit value when the points are taken densely on the curve, then the curve is said to have length, and this limit value is called the length of the curve.

A smooth curve has a length, which is:

For example, for a yen

We obtain the known value. Also, the length of the parabola y＝ ^x2 from x＝a to x＝b (a＜b) is

When the equation of a curve is given in the form F(x, y)＝0, if ∂F/∂x＝0, ∂F/∂y＝0 are true at a certain point, then in the vicinity of this point, F(x, y)＝0 generally cannot be expressed in the form x＝f(t), y＝g(t). This is called a singular point of the curve.

When the equation of a curve is given, tracing the outline of the curve is called tracing the curve. To do this, it is useful to investigate the range in which the curve exists, singular points, asymptote, etc.

A curve whose ends are connected, such as a circle, is called a closed curve. There are also closed curves that intersect with themselves, such as the lemniscate. A closed curve that does not intersect with itself is called a simple closed curve, or a Jordan closed curve. Jordan proved the following (1893): "A simple closed curve in a plane divides the plane into two parts, inside and outside, and no point inside can be connected to a point outside without intersecting with the curve." This is called Jordan's curve theorem. The content is common sense, but it is a difficult theorem to prove mathematically.

[Osamu Takenouchi]

"Various Curves" by Minoru Kurita (1966, Kyoritsu Publishing)

Curve length and closed curves
©Shogakukan ">

Curve length and closed curves

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

平面、あるいは空間内で、その点の座標が一つの実変数tの連続関数となっているものを曲線、または連続曲線という。平面内の曲線を平面曲線といい、方程式
x＝f(t), y＝g(t)
で表される。空間内の曲線を空間曲線といい、
x＝f(t), y＝g(t), z＝h(t)
で表される。

　曲線はまた、
F(x, y)＝0
あるいは
F(x, y, z)＝0, G(x, y, z)＝0
の形で与えられることもある。たとえば円は、
x＝r cos t, y＝r sin t
あるいは
x²＋y²＝r²
の形で与えられる。

　平面曲線はまた、y＝u(x)あるいは極座標によってr＝v(θ)で与えられることもある。以下では、おもに平面曲線を考える。

　「アステロイド」「カージオイド」「クロソイド」「コンコイド」「サイクロイド」「疾走線(しっそうせん)」「トラクトリックス」「螺線(らせん)」「リマソン」などの個々の曲線については、各項目を参照されたい。

［竹之内脩］

滑らかな曲線

平面曲線x＝f(t), y＝g(t)において、f(t), g(t)が導関数を有し、かつそれらが連続であるとき、この曲線を滑らかな曲線という。そして、f′(t), g′(t)を成分とするベクトル(f′(t), g′(t))を接ベクトルという。滑らかな曲線とは、接ベクトルが連続的に変わっていく曲線、という意味である。接ベクトルは、曲線上の近い2点
P(t)＝(f(t), g(t))
P(t＋Δt)＝(f(t＋Δt), g(t＋Δt))
を結ぶベクトルをΔtで割って極限をとったものである。

［竹之内脩］

曲線の長さ

2点A、Bを結ぶ曲線があるとき、この曲線上でA、Bの間に順に数多くの点
P₁, P₂,……, P_n-1 (A＝P₀, B＝P_n)
をとり、これらの点を次々と線分で結んで折れ線をつくる。この折れ線の長さ（各線分の長さの和）が、点のとり方をこの曲線上密になるようにしていったとき、ある極限値に収束するならば、この曲線は長さがあるといい、この極限値を曲線の長さという。

　滑らかな曲線は長さを有し、その長さは次のようになる。

たとえば、円の場合は

となり、周知の値を得る。また、放物線y＝x²のx＝aからx＝b(a＜b)までの長さは、

曲線の式がF(x, y)＝0の形で与えられているとき、もしもある点で∂F/∂x＝0,∂F/∂y＝0が成り立っていると、この点の近くでは一般にF(x, y)＝0をx＝f(t), y＝g(t)の形に表すことができない。これを、この曲線の特異点という。

　曲線の式が与えられたとき、この曲線の概形を描くことを、曲線の追跡という。このためには、曲線の存在する範囲や、特異点、漸近線(ぜんきんせん)などを調べるとよい。

　円のように両端がつながっている曲線を閉曲線という。レムニスケートのように自分自身と交わりをもつ閉曲線もある。自分自身と交わりをもたない閉曲線を単純閉曲線、あるいはジョルダン閉曲線という。ジョルダンは次のことを示した（1893）。「平面内の単純閉曲線は、平面を内部と外部の二つの部分に分け、内部の点と外部の点は、この曲線と交わることなしには結べない」。これをジョルダンの曲線定理という。内容は常識的であるが、数学的に証明しようとするとむずかしい定理である。

［竹之内脩］

『栗田稔著『いろいろな曲線』（1966・共立出版）』