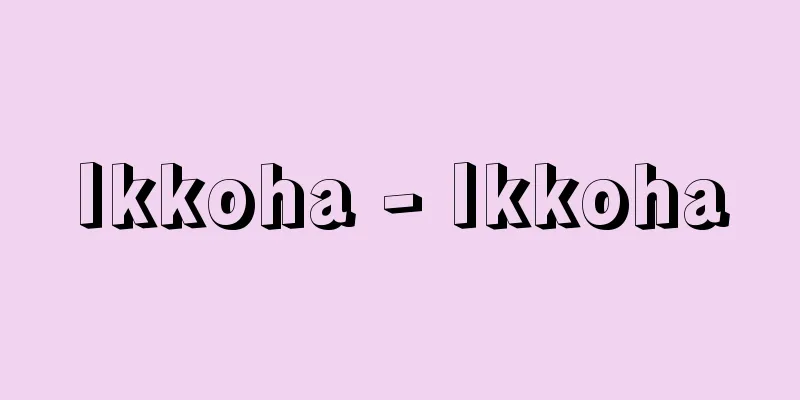Specific heat

Japanese: 比熱 - ひねつ（英語表記）specific heat

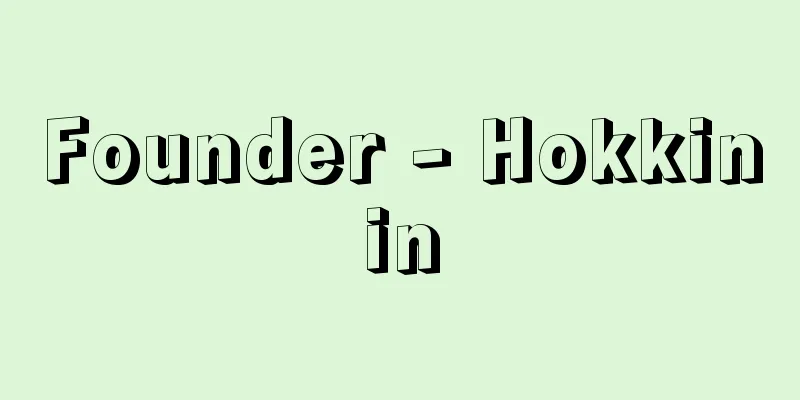
The amount of heat required to raise the temperature of 1 gram of a substance by 1 K. More generally, the amount of heat required to raise a certain amount of substance by 1 K is called heat capacity. It is equal to the specific heat multiplied by the number of grams of that substance. In other words, if the heat capacity of m grams of a substance is C and the specific heat of this substance is c , then C = mc . This article will focus on heat capacity.

The amount of heat required to raise the temperature of 1 gram of water by 1 K (strictly speaking, from 14.5°C to 15.5°C under 1 atmosphere) is 1 calorie (this is actually the definition of the calorie, abbreviated as calorie), so if the unit of heat is calorie, the specific heat of water is 1. The specific heat of a substance is almost always expressed in this way, as calorie/(gram-degree) (cal/g-K or cal-g ^- 1 K ^-1 ). For pure substances or gases, the heat capacity per mole (the amount of molecular weight multiplied by grams) is often used, and this is called the molar specific heat or molecular heat. If 1 mole is M , then the molecular heat C ^M is given by C ^M = M _c (for elements, this is called atomic heat).

The specific heat of materials used in machines and devices is often related to their performance. It is also well known that the extremely large specific heat of water makes the climate of areas close to the ocean mild. On the other hand, the quantity of specific heat is relatively easy to discuss even from an atomic perspective, and the degree to which the theoretical and experimental values of specific heat match is often a useful criterion for determining the validity of various theories in condensed matter physics.

Heat capacity varies depending on the conditions when the temperature of a substance is changed. What is often of interest is the difference between the heat capacity when the external pressure is kept constant, i.e., heat capacity at constant pressure (symbol C _P ), and the heat capacity when the volume of the sample is kept constant, i.e., heat capacity at constant volume (symbol C _V ). The only one that can be measured directly is the constant pressure heat capacity.

[Shozo Sawada]

Specific heat value

The specific heat at constant pressure of metals is around 0.03 to 0.2 cal/g・K, which is considerably smaller than the 1 of water. This is why metals heat up and cool down easily. On the other hand, the specific heat of hydrogen gas at room temperature is large, at 3.4. In any case, the specific heat of a substance is not a physical property that varies significantly depending on the type of substance, such as electrical conductivity.

[Shozo Sawada]

Thermodynamic expression for heat capacity

When an infinitesimal amount of heat d' Q is applied to a substance from the outside and the temperature of the substance changes from T to T + dT , the heat capacity C of the substance is, by definition, given by C = d' Q / dT . Meanwhile, according to the first law of thermodynamics, for an isotropic substance, dU = d' Q - pdV holds. Here, U , p , and V are the internal energy, pressure, and volume, respectively. Therefore, the heat capacity at constant volume is given by CV ₌ ( ∂U / ∂T ) ^V . Similarly, the heat capacity at constant pressure is given by CP ₌ ( ∂H / ∂T ) ^P . H is called enthalpy, and like U , is a thermodynamic potential (or thermodynamic function), and is expressed as H = U + pV . The difference between the heat capacity ^at constant pressure and the heat capacity _at constant volume is generally given by CP - _CV = TVα2 / ^κT . Here, α = (1/ V )(∂ V /∂ T ) ^P is the thermal expansion coefficient and κ ^T = -(1/ V )(∂ V /∂ p ) ^T is the isothermal compressibility. C _P is always > C _V , and this ratio, γ = C _P / C _V > 1, is a particularly important material constant for gases. For an ideal gas, from its equation of state pV = nm RT ( n _m is the number of moles and _R is the gas constant), it is easy to see that C _P - C _V = nm _R.

[Shozo Sawada]

Atomistic expression for heat capacity

(1) Ideal gas In a gas, the molecules that compose it fly about almost freely throughout the entire space occupied by the gas container, carrying thermal energy equivalent to the current temperature. The motion of these molecules can be adequately handled by classical mechanics (Newtonian mechanics). For example, the pressure of a gas is the force that corresponds to the change in momentum when a molecule collides with the wall of the container and bounces off. In particular, an ideal gas is one in which the size of the molecules can be ignored and there is no interaction between molecules (i.e. potential energy). In this case, it is easy to see that the energy (only kinetic energy) ε of one molecule is given by ε = (3/2) kT . Here, k = R / N _A is a very important fundamental constant called the Boltzmann constant, and N _A is the Avogadro constant, that is, the number of molecules in one mole of a substance. Since one molecule of an ideal gas has three degrees of freedom of motion, the above formula for ε indicates that the kinetic energy per degree of freedom is (1/2) kT . This law is called the law of equipartition of energy.

From the above, it can be easily said that ^the constant volume molecular heat capacity CVM of an ideal gas is _CVM ⁼ ₃ / 2R . Furthermore, from the above-mentioned equation for the relationship between the constant pressure heat capacity and the constant volume _heat capacity, CPM = 5/ 2R is obtained ^. Since R = 1.987cal/g・K, the theoretical value ^of the constant pressure molecular _{heat capacity CPM} is 4.97cal/g・K. On the other hand, for example, the measured value of the constant pressure specific heat of argon gas at room temperature is 0.124cal/g・K, so the measured value ^of CPM using a molecular weight of _39.95 is 4.95cal/mol・K, and these two values are in good agreement. Furthermore, the theoretical value of the ratio γ of the constant pressure specific heat and the constant volume specific heat is 5/3 = 1.67, but the measured value is also 1.67. In an inert gas such as argon gas, the molecules are atoms themselves, and the degree of freedom of the molecules is certainly three, so this result can be fully predicted. However, even for polyatomic gases such as _N2 and _CO2 , when the degree of freedom of rotation of the molecules is taken into account in addition to the degree of freedom of translation, the theoretical values _of CPM and γ also agree well with the measured values. These facts show that the above idea ^that gas is a collection of molecules that fly around almost freely is quite valid.

(2) Solids The measured values of the specific heat of solids near room temperature are closely related to the Dulong-Petit law (1819), which states that the molecular heat capacity of a solid is approximately 6 a cal/mol K, regardless of the type of solid. a is the number of atoms in a molecule, e.g., 1 for Cu and 2 for NaCl. This law is an extension of the law of equal distribution of kinetic energy described in the section on ideal gases, and can be easily explained. The extension law states that when potential energy exists in addition to kinetic energy, the energy per degree of freedom is kT . A solid is a system in which atoms are regularly arranged in a lattice, and each atom vibrates around its equilibrium position with thermal energy equivalent to the current temperature T (this is called lattice vibration). Vibrations generally occur due to the increase and decrease of kinetic energy and potential energy, so the energy per degree of freedom is kT . Since each atom has three degrees of freedom, the energy per atom is 3 kT , or 3 aRT per mole, and the molar heat capacity is 3 aR ≈6 a cal/mol・K.

In fact, Dulong-Petit's law only applies in a narrow temperature range around room temperature. The specific heat decreases as the temperature drops, and approaches zero rapidly near absolute zero. This significant temperature dependence of the specific heat at low temperatures could not be explained within the scope of classical physics, and was only explained by quantum theory. That is, a vibrating atom can be considered as a harmonic oscillator, but while the energy it can take is continuous in classical theory, it is discrete in quantum theory, such as 3nhν ( n = 0, 1, 2, ...). Here, ν is the frequency, and h is called the Planck constant, the most important fundamental constant in quantum theory. Due to the discreteness of this energy level, statistical mechanics clearly shows that the specific heat decreases significantly at low temperatures.

[Shozo Sawada]

Anomalous specific heat

The specific heat of a substance usually increases monotonically with increasing temperature. However, it is not rare for the specific heat to show an anomalous temperature dependence, as in the case of potassium dihydrogen phosphate. This type of specific heat is called anomalous specific heat, and almost always occurs as a result of a phase transition. A phase transition is a phenomenon in which the properties of a substance suddenly change across a temperature (transition point) that is specific to that substance. Since the unique properties of substances, such as ferromagnetism, ferroelectricity, and superconductivity, appear as a result of a phase transition, phase transitions are a phenomenon of great interest in both fundamental and applied fields.

[Shozo Sawada]

"Basic Physics 2" by Masami Sawada, Seizaburo Kageyama, Takehiko Oguchi, and Taketoshi Yanagi (1973, Maki Shoten)

[Reference] | Equipartition of energy | Kinematic theory of gases | Atomic heat | Phase transition

Normal temperature dependence
Reduced temperature dependence of constant-volume molecular heat capacity according to Debye's theory. Experimental values for copper (Cu) and silver (Ag) are also shown. ©Shogakukan ">

Normal temperature dependence

Anomalous temperature dependence (anomalous specific heat)
The temperature dependence of the measured constant pressure molecular heat capacity of potassium dihydrogen phosphate (KDPO) ©Shogakukan ">

Anomalous temperature dependence (anomalous specific heat)

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

1グラムの物質の温度を1K上げるのに必要な熱量。より一般的には、ある一定量の物質を1K上げるのに必要な熱量のことを熱容量heat capacityという。比熱にその物質のグラム数を乗じたものに等しい。すなわちmグラムの物質の熱容量をC、この物質の比熱をcとすると、C＝mcである。本項では熱容量を中心として記述する。

　水1グラムの温度を1K（厳密には1気圧下で14.5℃から15.5℃）上げるのに必要な熱量は1カロリー（これが実はカロリーcalorie〈略号cal〉の定義）であるから、熱量の単位をカロリーととると、水の比熱は1である。物質の比熱はほとんどいつもこのように、カロリー／（グラム・度）で表される（cal/g・Kあるいはcal・g^-1K^-1である）。純物質あるいは気体に対しては1モル（分子量にグラムをつけた量）に対する熱容量を用いることが多く、これをモル比熱molar heatまたは分子熱molecular heatという。1モルをMとすると分子熱C^Mは、C^M＝M_cで与えられる（元素の場合には、これは原子熱とよばれる）。

　機械、装置などに使用されている材料の比熱が、それらの性能に関係することも少なくない。また、水の比熱が非常に大きいことが、海洋に近い地域の気候を温和にしていることはよく知られたことである。一方、比熱という量は原子論的にも比較的議論しやすいものであって、物性論におけるいろいろの理論の正当性を判断するのに、比熱の理論値と実験値とがどの程度あうかということが、有用な判定資料になることが多い。

　熱容量は、物質の温度を変えるときの条件によって異なる。よく関心がもたれるのは、外からの圧力が一定に保たれる場合の熱容量すなわち定圧熱容量heat capacity at constant pressure（記号C_P）と、試料の体積が一定に保たれる場合の熱容量すなわち定積熱容量heat capacity at constant volume（記号C_V）との差異である。直接に測定できるものは定圧熱容量である。

［沢田正三］

比熱の値

金属の定圧比熱は0.03～0.2cal/g・K程度で、水の1に比しては相当小さい。これが金属が「熱しやすく冷めやすい」ゆえんである。一方、水素ガスは室温で3.4と大きい。いずれにしても物質の比熱は、電気伝導率などのように物質の種類によって著しく差異のあるような物性量ではない。

［沢田正三］

熱容量の熱力学的表式

物質に外部から微小な熱量d'Qが加えられて、物質の温度がTからT＋dTに変化するとき、物質の熱容量Cは定義によりC＝d'Q／dTで与えられる。一方、熱力学第一法則により、等方性物質ではdU＝d'Q－pdVが成り立つ。ここでU、p、Vはそれぞれ内部エネルギー、圧力、体積である。したがって定積熱容量はC_V＝(∂U/∂T)^Vで与えられる。また、定圧熱容量はC_P＝(∂H/∂T)^Pで与えられる。Hはエンタルピーとよばれ、Uと同じく一つの熱力学的ポテンシャル（あるいは熱力学的関数）であって、H＝U＋pVで表される。定圧熱容量と定積熱容量との差は一般にはC_P－C_V＝TVα²/κ^Tで与えられる。ここにα＝(1/V)(∂V/∂T)^Pは体熱膨張率、κ^T＝－(1/V)(∂V/∂p)^Tは等温圧縮率である。つねにC_P＞C_Vであって、その比すなわちγ＝C_P/C_V＞1は気体ではとくに重要な物質定数である。理想気体ではその状態方程式pV＝n_mRT（n_mはモル数、Rは気体定数）から、C_P－C_V＝n_mRであることが容易にわかる。

［沢田正三］

熱容量の原子論的表式

(1)理想気体　気体ではそれを構成する分子が、現在の温度に相当する熱エネルギーをもって、ほとんど自由にこの気体の容器が占めている全空間を飛び回っている。これらの分子の運動のようすは古典力学（ニュートン力学）で十分取り扱える。たとえば、気体の圧力は、分子が容器の壁に衝突して跳ね返るときの運動量の変化に対応する力である。とくに理想気体は、分子の大きさを無視することができ、かつ分子の間の相互作用（すなわちポテンシャルエネルギー）がまったくないものである。この場合には、分子1個のもつエネルギー（運動エネルギーだけ）εはε＝(3/2)kTで与えられることがすぐいえる。ここに、k＝R/N_Aはボルツマン定数とよばれるたいへん重要な基本定数であり、N_Aはアボガドロ定数、すなわち物質1モル中の分子の数である。理想気体の1個の分子の運動の自由度は3であるから、先のεの式は、各自由度当りの運動エネルギーは(1/2)kTであることを示す。この法則はエネルギー等分配則とよばれる。

　理想気体の定積分子熱容量C_V^Mは以上のことからすぐにC_V^M＝3/2Rであることがいえる。また前述の定圧熱容量と定積熱容量の関係式から、C_P^M＝5/2Rが得られる。R＝1.987cal/g・Kであるから、定圧分子熱容量C_P^Mの理論値は4.97cal/g・Kである。一方、たとえば、アルゴンガスの定圧比熱の実測値は、室温で0.124cal/g・Kであるから、分子量39.95を用いてC_P^Mの実測値は4.95cal/mol・Kであって、この両者はよく一致する。さらに定圧比熱と定積比熱の比γの理論値は5/3＝1.67であるが、実測値も1.67である。アルゴンガスのような不活性ガスでは、分子は原子そのもので、分子の自由度は確かに3であるから、この結果は十分予測できる。ところが、N₂やCO₂のような多原子分子気体でも、分子の並進の自由度のほかに回転の自由度を考慮すると、C_P^M、γの理論値と実測値とがやはりよく一致する。これらのことは、気体はほとんど自由に飛び回っている分子の集まりであるとする以上の考え方がまことに妥当であることを示すものである。

(2)固体　固体の室温付近の比熱の実測値に関しては、固体の分子熱容量が固体の種類に無関係に、約6acal/mol・Kであるというデュロン‐プチの法則（1819）がよく当てはまる。aは、分子中の原子の数であり、たとえばCuでは1、NaClでは2である。この法則は、理想気体のところで述べた運動エネルギーに関する等配則の拡張されたもので容易に説明できる。拡張則は、運動エネルギーのほかにポテンシャルエネルギーが存在する場合には、1自由度当りのエネルギーはkTであるということである。固体は原子が規則正しく格子状に配列したものであるが、各原子はその平衡位置の周りで現在の温度Tに相当する熱エネルギーをもって振動（これは格子振動といわれる）をしている。振動は、一般に運動エネルギーと位置のエネルギーとの消長でおこるものであるから、1自由度当りのエネルギーはkTである。原子1個の自由度は3であるから、原子1個当りのエネルギーは3kTであり、1モル当りでは3aRTで、分子熱容量は3aR≈6acal/mol・Kなのである。

　デュロン‐プチの法則は、実は室温付近の狭い温度範囲でしか成立しない。比熱は、温度降下とともに減少し、絶対零度付近では急激に0に近づく。比熱の低温におけるこのような顕著な温度依存性は、古典物理学の範囲内では説明できず、量子論によって初めて説明できた。すなわち、振動している原子は調和振動子と考えてよいが、そのとりうるエネルギーが、古典論では連続的なのに対して、量子論では3nhν（n＝0,1,2,……）のようにとびとびである。ここに、νは振動数であり、hはプランク定数とよばれ、量子論におけるもっとも重要な基本定数である。このエネルギー準位の離散性のために、比熱が低温で著しく減少することが統計力学で明瞭(めいりょう)に示される。

［沢田正三］

異常比熱

物質の比熱は、温度上昇とともに、普通は単調に増加する。ところが、リン酸二重水素カリウムのように、比熱が異常な温度依存性を示すことも少なくはない。このような比熱は、異常比熱anomalous specific heatといわれ、ほとんど相転移に伴っておこる。相転移とは、ある物質の性質が、その物質に特有な温度（転移点）を境として急に変化する現象である。強磁性、強誘電性、超伝導性などの物質の特異な性質は相転移に伴って現れるので、相転移は基礎分野でも応用分野でも大いに関心がもたれる現象である。

［沢田正三］

『沢田正三・影山誠三郎・小口武彦・柳武敏著『基礎物理2』（1973・槇書店）』

[参照項目] | エネルギー等分配則 | 気体分子運動論 | 原子熱 | 相転移

普通の温度依存性

異常な温度依存性（異常比熱）

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例

<<: Hinenosho

>>: Vinegar - vinegar