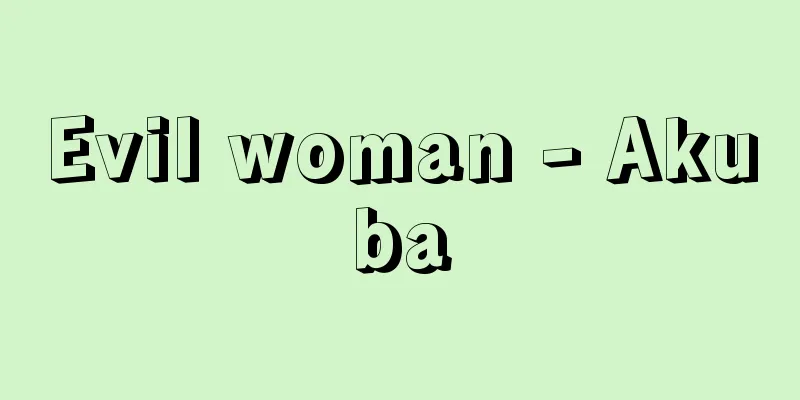Lebesgue integral - Lebesgue integral

The integrals that usually appear in calculus textbooks are called Riemann integrals, and although the definition is simple, they have some shortcomings. For example, even if an integrable sequence of functions {f _n (x)} converges to f(x) at each point x, f(x) may not be integrable, or

Also, when changing the order of integration of a function of two variables f(x,y), conditions such as continuity that are unrelated to integration may be required.

In his doctoral thesis of 1902, Lebesgue constructed an integral that eliminates these shortcomings and yet gives the same integral value for all Riemann integrable functions. This is called the Lebesgue integral, and is an essential part of the foundations of modern analysis. However, the method of constructing the Lebesgue integral is somewhat complicated, and there are various styles of improving it, but they all result in the same integral.

Abstractly, consider a measure space (X,M,m), i.e., a family M of subsets of a set X, where a measure m(E) is defined for a set E∈M, and this measure is completely additive. The sets that are elements of M are called measurable sets.

A real-valued function f(x) on X is a set {x∈X;f(x)＞α}∈M (a measurable set) for any real number α.
When this happens, the function is called a measurable function. For a measurable function f(x),

Then, since E _k,n ∈M,

When is determined, the function f(x) is integrable. This limit value is called the Lebesgue integral of the function f(x) on the measurement space (X,M,m),

The set of integrable functions is denoted by L ¹ (X,M,m) or simply L ^1. In particular, if X is an n-dimensional Euclidean space R ⁿ and E is an interval, and m is the volume |E| of the interval E, then

This is expressed as:

Some typical properties of the Lebesgue integral are:
(1) If a function f(x) is measurable and there is an integrable function g(x) such that |f(x)|≦g(x), then f(x) is also integrable.

(2) If {f _n (x)} ⊂ ^{L 1} (X,M,m), f _n (x) → f(x), and |f _n (x)|≦g(x)∈L ¹ (X,M,m), then f(x)∈L ¹ (X,M,m), and

This shows that integrals and lim commute, which is called the Lebesgue convergence theorem.

(3) If f(x,y)∈L ¹ (X,M,m) is a function of two variables, then the multiple integral becomes a repeated integral,

(4) A measurable function f(x) on the measure space (X, M, m),

Let L ^p (X,M,m) be the set of functions f(x) such that

Then L ^p (X,M,m) is a Banach space. In particular, when p=2, it is a Hilbert space (Riesz-Fisher theorem).

In this way, the Lebesgue integral created a function space that is useful for functional analysis (defining the norm of a Riemann integrable function using the formula (*) does not make it complete).

[Haruo Sunouchi]

``Introduction to Lebesgue Integral'' by Seizo Ito (1963, Shokabo) ▽ ``Introduction to Lebesgue Integral'' by Haruo Sunouchi (1974, Uchida Rokakupa)''

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

普通、微積分学の教科書に出てくる積分はリーマン積分とよばれるもので、定義は簡単であるが、いくつかの欠点をもっている。たとえば、積分可能な関数列｛f_n(x)｝が、各点xでf(x)に収束しても、f(x)が積分可能でなかったり、

に収束しなかったりすることである。また、二変数の関数f(x,y)の積分順序の変換に、積分と関係のない連続性などの条件が必要になったりする。

　ルベーグは1902年の学位論文で、これらの欠点を除き、しかも、リーマン積分可能な関数の積分の値は同じになるような積分を構成した。これがルベーグ積分とよばれているもので、近代解析学の基礎として不可欠のものとなっている。ただし、ルベーグ積分の構成法はやや複雑であり、いろいろ改良した流儀があるが、いずれも同じ積分に帰着する。

　抽象的に、測度空間(X,M,m)を考える。すなわち、集合Xの部分集合の族Mで、集合E∈Mには測度m(E)が定義され、これが完全加法的な測度になっているとする。このときMの要素である集合を可測集合という。

　X上の実数値関数f(x)が、任意の実数αに対し、集合
　　｛x∈X;f(x)＞α｝∈M（可測集合）
となるとき、この関数を可測関数という。可測関数f(x)に対し、

と置けば、E_k,n∈Mであるから

が確定するとき、関数f(x)は積分可能となる。この極限値を関数f(x)の測定空間(X,M,m)上のルベーグ積分といい、

で表す。また、積分可能な関数の集合をL¹(X,M,m)または簡単にL¹で表す。とくにXがn次元ユークリッド空間Rⁿで、Eが区間のとき、mが区間Eの体積|E|を表すならば、

と表す。

　ルベーグ積分の代表的な特性をいくつかあげると、
（1）関数f(x)が可測で、ある積分可能な関数g(x)があって、|f(x)|≦g(x)ならば、f(x)も積分可能となる。

（2）｛f_n(x)｝⊂L¹(X,M,m),f_n(x)→f(x)、しかも|f_n(x)|≦g(x)∈L¹(X,M,m)ならばf(x)∈L¹(X,M,m)となり、

これは積分とlimが交換可能であることを示している。これをルベーグの収束定理という。

（3）二変数の関数f(x,y)∈L¹(X,M,m)ならば、重積分は繰り返し積分となり、

（4）測度空間(X,M,m)上の可測関数f(x)で、

となる関数f(x)の集合をL^p(X,M,m)とし、これにノルムを

で定義すると、L^p(X,M,m)はバナッハ空間となる。とくに、p=2のときはヒルベルト空間である（リース‐フィッシャーの定理）。

　このように、ルベーグ積分によって関数解析に役だつ関数空間がつくられた（リーマン積分可能な関数に、リーマン積分により、式（＊）でノルムを定義しても完備にはならない）。

［洲之内治男］

『伊藤清三著『ルベーグ積分入門』（1963・裳華房）』▽『洲之内治男著『ルベーグ積分入門』（1974・内田老鶴圃）』

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例