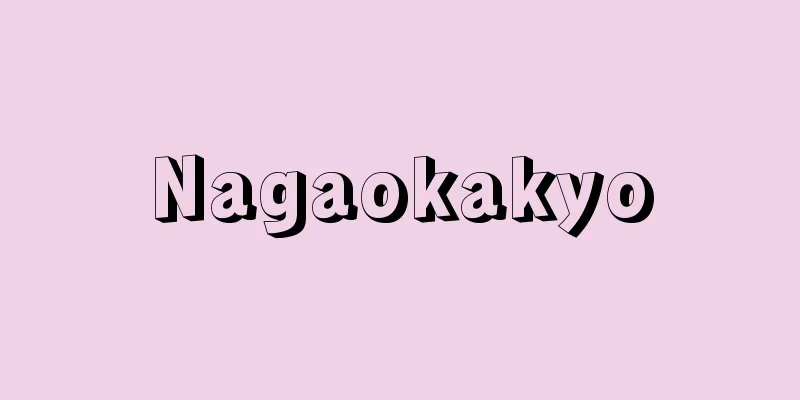Exploded view - Tenkaizu

Transferring the surface of a solid onto a plane without changing the quantities of that surface (length, angle, etc.) is called development (congruent transformation), and the transferred plane figure is called a development diagram. In the case of a polyhedron whose faces are flat, a perfect development diagram can be obtained, but this is not necessarily the case for curved surfaces. A surface for which a perfect development diagram is possible is called a developable surface. This is a limited type of ruled surface, which is a curved surface created by moving a straight line (generator), and simple curved surfaces fall into this category. Typical examples include cylinders, cones, and quasi-spiral surfaces (surfaces formed by the tangents of a spiral). When it comes to curved surfaces, a developable surface only means that it is possible in principle, and the development is usually found by an approximation method.

The development of a polyhedron is represented as a series of polygons. The development of a right circular cylinder consists of a rectangle on the sides and two circles on the base. The development of a right circular cone consists of a sector on the sides and a circle on the base.

For example, to create an oblique cone, the circumference of the base circle is divided equally, and a polygonal pyramid is assumed with the vertices of the equally divided points and the vertex of the oblique cone as its vertices. It is easy to develop a polygonal pyramid, since the actual length of the edges and the actual shape of the faces are easy to determine. In this case, if the number of equally divided points is increased, the polygonal pyramid can approximate a circular cone, and a more approximate development diagram can be obtained. This is called the radial method due to the shape of the development diagram.

Like cylinders, it is easy to obtain a development diagram for a cone, so one method is to approximate a non-developable surface with these, and then further develop it into an approximate polyhedron. However, the most common method is to approximate a polyhedron made up of small triangles created by connecting points on the surface. This is called the triangulation method.

There are various purposes for creating development drawings (for example, interior development drawings for architecture), but one of the most important is the problem of geodesic lines. A geodesic line is a line that shows the shortest distance between two points on a surface, but on the development drawing of that surface, this line is a straight line. Therefore, a geodesic line can be obtained by determining the positions of two points on the development drawing, connecting them with a straight line, and then transferring each point on this straight line onto the surface when returning the development drawing to its original surface.

[Yoshio Tamakoshi]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

立体の面をその面の量（長さ・角度など）を変えずに平面上に移すこと（合同変換）を展開といい、その移された平面図形を展開図という。面が平面である多面体の場合には完全な展開図が得られるが、曲面の場合はかならずしもそうではない。完全な展開図の可能な面を可展面という。それは直線（母線）の移動によってできる曲面である線織面のなかでも限られたもので、単曲面が該当する。円柱・円錐(えんすい)・類似螺旋(らせん)面（螺線の接線により構成される面）などがその代表例である。曲面では、可展面といっても、原理的に可能だということであって、展開法は近似法によって求めるのが普通である。

　多面体の展開図は多角形のつながりとして表される。直円柱の展開図は側面からできる長方形と二つの底面の円からできている。また、直円錐の展開図は側面からできる扇形と底面の円からできている。

　たとえば、斜円錐では、底円の円周を等分し、その等分点と斜円錐の頂点をおのおの頂点とする多角錐を仮定する。多角錐の展開は、稜(りょう)の実長、面の実形が求めやすいので、容易である。その際、等分点の数を増せば、多角錐は円錐に近似しうるので、より近似した展開図が得られる。これは展開図の形から放射線法といわれる。

　このように円錐は、円柱と同様、展開図が得られやすいので、可展面でない曲面をその部分ごとにこれらで近似させ、それをさらに多面体で近似展開する方法がある。しかし、一般的には、曲面上の点を結んでできる小三角形よりなる多面体に近似する方法がとられる。三角形法という。

　展開図の作成目的は種々（たとえば建築での室内展開図など）あるが、そのなかで重要なものとして測地線の問題がある。測地線とは、面上の2点間の最短距離を示す線であるが、その面の展開図上では、その線は直線となる。したがって、2点の展開図上の位置を求め、それを直線で結び、展開図を元の面に戻す際にこの直線上の各点を面上に移すことで、測地線が求められる。

［玉腰芳夫］

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例