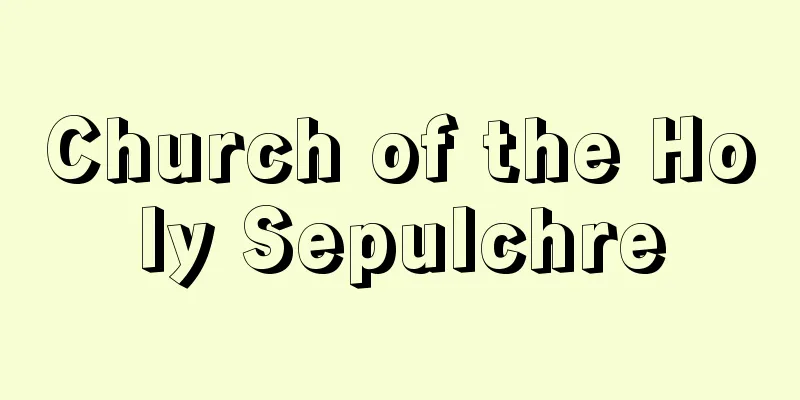
Line integral

Japanese: 線積分 - せんせきぶん

This is an integral along a curve, also known as a curve integral. Let L be a curve with a certain length that connects two points A and B. The line integral of a function f(P) on L

Define as follows. Take a number of points P ₁ , P ₂ , …, P _n-1 (A=P ₀ , B=P _n ) on the curve between A and B, and take point Q ₁ between P ₀ and P ₁ , point Q ₂ between P ₁ and P ₂ , …, point Q _n between P _n-1 and P _n ( Figure A ). Then,

Consider the following. Here, it is the length of the line segment connecting P _k-1 ,P _k . When the points P ₁ ,P ₂ ,……,P _n-1 ,Q ₁ ,Q ₂ ,……,Q _n are chosen in various ways, and no matter how they are chosen, when these points are densely arranged on L, if they converge to a certain limit value, then this limit value is defined as the value of the line integral (*) mentioned above. If f(P) is bounded and continuous in a part of the plane that includes L, then the line integral exists. In the approximate sum of the line integral, replace with, for example, the difference in the x-coordinates of each point, x _k -x _k-1 , and we get

Consider the limit value of this, the line integral

Similarly, the line integral

For example, if the area of a part enclosed by a simple closed curve L on a plane ( Figure B ) is S, then

Suppose the boundary of a bounded region D on a plane consists of a finite number of piecewise smooth simple closed curves _C1 , _C2 , ..., _Cm ( Figure C ). All of these boundary curves are oriented while looking leftward inside D, and are collectively represented by C. If f(x,y) and g(x,y) are C ^first- class functions (functions with continuous partial derivatives) within the range including the boundary of D, then the following equation holds.

This is called Green's theorem.

[Osamu Takenouchi]

Line integral of a complex function

In the approximate sum of line integrals, we replace with the complex difference z _k -z _k-1 representing each point.

(Z _k ′ is the complex number corresponding to Q _k ), and the limit value of this is

is defined. Cauchy's integral theorem

and Cauchy's integral formula

etc. are expressions using this line integral.

[Osamu Takenouchi]

Line integral diagram (Figure A)
©Shogakukan ">

Line integral diagram (Figure A)

Line integral diagram (Figure B)
©Shogakukan ">

Line integral diagram (Figure B)

Line integral diagram (Fig. C)
©Shogakukan ">

Line integral diagram (Fig. C)

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

曲線に沿って行う積分のことで、曲線積分ともいう。いま、Lは2点A、Bを結ぶ長さのある曲線とする。L上での関数f(P)の線積分

を次のように定める。A、Bの間の曲線の部分に順に数多くの点P₁,P₂,……,P_n-1（A=P₀,B=P_nとする）をとり、またP₀,P₁の間に点Q₁、P₁,P₂の間に点Q₂、……、P_n-1,P_nの間に点Q_nをとり（図A）、和

を考える。ここでは、P_k-1,P_kを結ぶ線分の長さである。点P₁,P₂,……,P_n-1,Q₁,Q₂,……,Q_nのとり方をいろいろに変えるとき、どのようにそれらをとっても、これらの点をL上密になるようにしていったとき、ある極限値に収束するならば、この極限値を前記線積分（＊）の値と定義する。f(P)がLを含むような平面のある部分において有界連続ならば、線積分は存在する。線積分の近似和において、を、たとえば、各点のx座標の差x_k-x_k-1で置き換えて、

を考え、これの極限値として線積分

が定義される。同様に、線積分

も定められる。たとえば、平面上の長さのある単純閉曲線Lで囲まれた部分（図B）の面積をSとすれば、

　平面上の有界な領域Dの境界が、区分的に滑らかな有限個の単純閉曲線C₁,C₂,……,C_mからなるとする（図C）。これらの境界の曲線は、すべてDの内部を左側に見ながら回る向きをつけてあるものとし、まとめてCで表す。いま、f(x,y),g(x,y)が、D上境界も含めた範囲でC¹級関数（連続な偏導関数を有する関数）ならば、次の式が成立する。