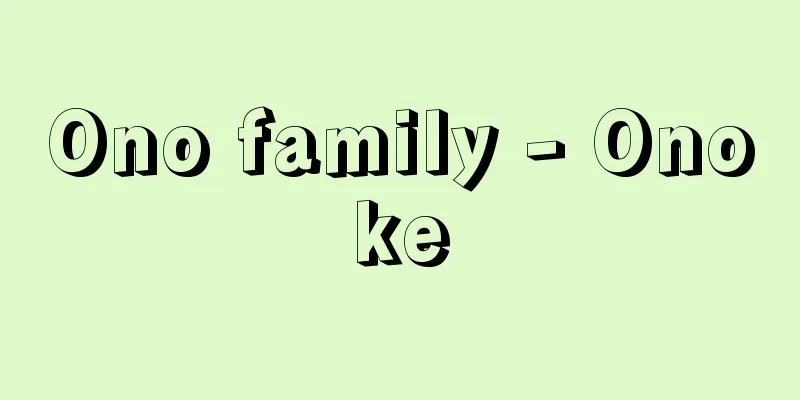Tangent - tangent

|
If there is a line connecting point P0 on a curve to a nearby point P, and we consider the limit as P approaches P0 , if this line P0P approaches a certain line l, then this line l is called the tangent to the curve at point P0 . Tangent lines used to be written as intercept lines, but this term is no longer used. The equation of the tangent at point P 0 (x 0 ,y 0 ) on a quadratic curve such as an ellipse, hyperbola, or parabola is y=m(xx 0 )+y 0 [Osamu Takenouchi] ©Shogakukan "> Tangent line explanation diagram Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend |
|
曲線上の点P0と、その近くの点Pとを結んだ直線があり、PをP0に近づけた極限を考えるとき、この直線P0Pが、ある一定の直線lに近づくならば、この直線lを、この曲線上の点P0における接線という。接線は、切線と書いたこともあったが、いまはこの字は使わない。 楕円(だえん)、双曲線、放物線などの二次曲線の曲線上の点P0(x0,y0)における接線の式を [竹之内脩] ©Shogakukan"> 接線説明図 出典 小学館 日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について 情報 | 凡例 |
<<: Snow line - Sessen (English spelling) snowline
Recommend
Dai Viet (English spelling)
The name of the country of Vietnam used from 1054 ...
Prunus × parvifolia
…Cherry blossoms have been loved by Japanese peop...
War of Austrian Succession
An international war over the succession to the A...
Orochi tribe - Orochi tribe (English spelling)
A Tungusic ethnic group living in the basins of th...
Otome Pass - Otome Pass
...The pass offers a good view of Mt. Fuji and th...
Cosmos Satellite
A series of artificial satellites from the former ...
direct lift control
...This is called relaxed static stability. (3) D...
Spiral Nebula Theory
...However, this Russell-Littleton encounter theo...
Thyroptera discifera (English spelling) Thyropteradiscifera
...A mammal belonging to the family Thyropteridae...
Molecular beam epitaxy
...Since around 1970, development has progressed ...
maphrian
…the title of bishop second only to patriarch in ...
marché (English spelling) marche
…As a result, the artisans tried to create an org...
Micro‐whip‐scorpion (paper-twisting worm)
A small arthropod belonging to the order Palpigrad...
Denim - Denim (English spelling)
Generally, it is a thick cotton fabric with a thr...
Kagetsu (Kagetsu) - Crassula portulacea; baby jade; cauliflower ears
A succulent plant of the Crassulaceae family. It i...