Regular polygon

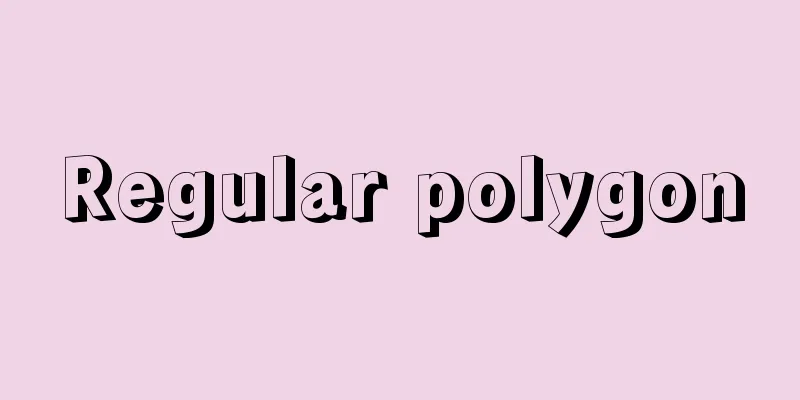
A regular polygon is a polygon in which all sides are equal and all interior angles are equal. A regular polygon is also a polygon made by connecting the points that divide a circle equally. The center of this circle is called the center of the regular polygon. This circle is the circumscribing circle of the regular polygon. It is also possible to draw an inscribing circle around a regular polygon with its center as its center. A regular polygon with n sides, and therefore n vertices, is called a regular n-polygon. A regular quadrilateral is usually called a square. A regular n-polygon can be divided into n isosceles triangles by connecting the ends of each side to the center. The apex angle is 1/n of 360 degrees. A regular n-polygon overlaps itself by rotating 360 degrees by n around its center. In other words, a regular n-polygon is a figure with 360-degree rotational symmetry by n. When n is an even number, it is a figure with point symmetry with respect to the center, that is, 180-degree rotational symmetry. All regular n-polygons have n axes of line symmetry. When n is an odd number, the axes of symmetry are the perpendicular bisectors of the n sides, each of which passes through one vertex. When n is an even number, the axes of symmetry are the perpendicular bisectors of each side and the straight lines connecting the opposite vertices.

Constructing a regular n-gon is nothing more than dividing the circumference of a circle into n equal parts. This is called the Circumference Division Problem. It is well known that C. F. Gauss succeeded in constructing a regular 17-gon when he was 19 years old. The Circumference Division Problem was subsequently completely solved by Gauss. As a result, it was made clear that when n is 7, 9, 11, 13, 14, 18, etc., it is not possible to divide the circumference of a circle into n equal parts using a ruler and compass.

A plane can be filled with any one of the following tiles: equilateral triangles, squares, or regular hexagons. These are the only three types of tiles that can be tiled with a single type of regular polygon. There are various types of tiling that use two or more types of regular polygons, and 11 types are known that have uniform convergence at each vertex.

[Toshio Shibata]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

すべての辺が等しく、すべての内角も等しい多角形を正多角形という。正多角形は、円周を等分した点を順々に結んでできる多角形でもある。この円の中心を正多角形の中心という。この円は正多角形の外接円である。また正多角形には、その中心を中心として内接円をかくこともできる。辺の個数、したがって頂点の個数がnである正多角形を正n角形という。正四角形を普通、正方形という。正n角形は、各辺の両端と中心とを結んでn個の二等辺三角形に分けられる。その頂角は360度のn分の1である。正n角形は、その中心の周りのn分の360度の回転によって自分自身に重なる。すなわち、正n角形はn分の360度回転対称な図形である。nが偶数のときはその中心に関して点対称、すなわち180度回転対称な図形である。正n角形はすべてn個の線対称の軸をもっている。その対称軸は、nが奇数のときはn個の辺の垂直二等分線で、それぞれ一つの頂点を通る。nが偶数のとき線対称の軸は各辺の垂直二等分線および相対する頂点を結ぶ直線である。

　正n角形の作図は、円周をn等分することにほかならない。これを円周等分問題という。C・F・ガウスが19歳のとき正17角形の作図に成功したことは有名。円周等分問題はその後ガウスにより完全に解決された。その結果、nが7、9、11、13、14、18などの場合は、定規(じょうぎ)とコンパスで円周を等分することはできないことが明らかにされた。

　正三角形、正方形、正六角形のタイルのうち、どれか1種類を用いて平面を埋め尽くすことができる。1種類の正多角形でタイル張りができるのはこの三つだけである。2種類以上の正多角形によるタイル張りはいろいろとあり、各頂点に集まるようすが一様なものは11種あることが知られている。

［柴田敏男］

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例