Limit - limit

Japanese: 極限 - きょくげん

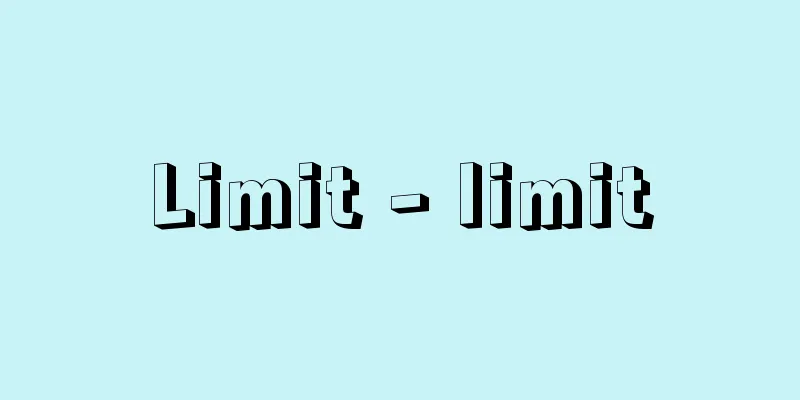
In a sequence of numbers a ₁ , a ₂ ,……, a _n ,……, if the index n of a _n becomes infinitesimally large and approaches a certain value A, then a _n is said to converge to A, and A is called the limit value of this sequence.

Also, when n is increased, if a _n becomes infinitely large, it is said that a _n diverges to positive infinity,

When n is increased, if a _n is negative and its absolute value becomes infinitely large, a _n is said to diverge to negative infinity,

When _{a n} converges to a limit value or diverges to positive or negative infinity, it is said to have a limit; when it does not, it is said to have no limit. The limit of a sequence is thus a conceptual term for thinking about what a _n will be like when n becomes infinitely large in a sequence.

[Osamu Takenouchi]

Limit of a function

Suppose the function f(x) is defined near x = a (it does not have to be defined at x = a). In this case, the limit problem is what happens to the value of f(x) as x approaches a infinitesimally close to a.

(1) f(x) approaches a certain fixed value b infinitely. At this time, f(x) is said to converge to b, and b is called the limit value of f(x) when x approaches a.

This can be written as (1) in the figure .

(2) f(x) becomes larger than any value. At this point, f(x) is said to diverge to positive infinity,

( Figure (2)). When f(x) takes a negative value and its absolute value becomes infinitely large, f(x) is said to diverge to negative infinity. The above is the case where there is a limit. Next, we consider the case where there is no limit.

(3) f(x) is bounded (a function is said to be bounded when its value does not exceed a certain number and does not become smaller than a certain number), but it has no limit ((3) in the figure ).

(4) f(x) is unbounded and has no limit ((4) in the figure ).

In addition, when there is no limit, there may be a limit when looking at only one side of a ((5) and (6) in the figure ). When there is a limit value when approaching from the left side, this is called the left-side limit,

Similarly, the right-side limit value

is determined.

[Osamu Takenouchi]

[Reference] | Convergence | Sequence

Limit (limit of a function) [Diagram]
©Shogakukan ">

Limit (limit of a function) [Diagram]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

数列a₁, a₂,……, a_n,……において、添数のnが限りなく大きくなるとき、a_nの値がある値Aに限りなく近づくならば、a_nはAに収束するといい、Aをこの数列の極限値limit valueといって、

と書く。また、nを大きくするとき、a_nが限りなく大きくなるならば、a_nは正の無限大に発散するといい、

と書く。nを大きくするとき、a_nが負でその絶対値が限りなく大きくなるならば、a_nは負の無限大に発散するといい、

と書く。a_nがある極限値に収束する場合、あるいは正または負の無限大に発散するとき、極限があるといい、そうでないとき、極限がないという。数列の極限はこのように、数列においてnが限りなく大きくなるとき、a_nがどのようになるかを考えるための概念的な用語である。