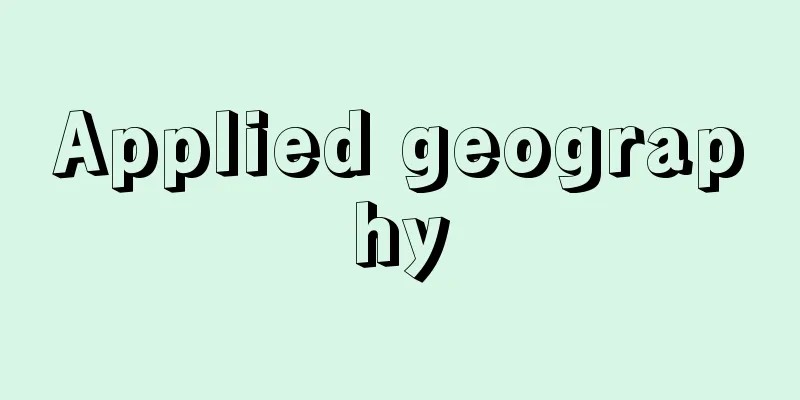Curvilinear coordinates

Japanese: 曲線座標 - きょくせんざひょう（英語表記）curvilinear coordinates

Coordinates based on a family of curves. _Let the Cartesian coordinates in three-dimensional space be ( x , y , z ), and the three functions _be _ξ1 = f1 ( x , y , z ), _ξ2 = f2 ( x , y , z ), and _ξ3 ₌ f3 ( x _, y , z ). Now _, _let c1 , c2 _, and c3 be constants, respectively, and let _ξ1 = c1 , _ξ2 ₌ c2 , and _ξ3 = c3 _, all of which represent curved surfaces. By changing _the values _of c1 , c2 , and c3 , three _types of surfaces can be obtained. When one surface is taken from each of these three groups of surfaces, they intersect at a single point P. Conversely, there is a unique set of surfaces _ξ1 = c1 , _ξ2 = c2 , _ξ3 = c3 that pass through any point in space. In this way, there is a _one - _{to-one correspondence} between each point ( x , y , z ) in space and the set of real numbers ( _ξ1 , _ξ2 , _ξ3 ). In other words, the set of real numbers ( _ξ1 , _ξ2 , _ξ3 ) can be considered as the coordinates that define _the point ( x , y , z ) in space. These ( _ξ1 , _ξ2 , _ξ3 ) are called the curvilinear coordinates around point P in three-dimensional space. _{The necessary} _{and sufficient condition for the three functions f1} ₍ x , y , z ), f2 ( x , y , z ), and f3 ( x , y , z ) to define the curvilinear coordinates ( _ξ1 , _ξ2 , _ξ3 ) is that the Jacobian (function determinant) is not 0. The same is true for two-dimensional and four-dimensional or higher spaces.

Source: Encyclopaedia Britannica Concise Encyclopedia About Encyclopaedia Britannica Concise Encyclopedia Information

Japanese:

曲線族による座標。3次元空間の直交座標を (x，y，z) とし，3つの関数を ξ₁＝f₁(x，y，z)， ξ₂＝f₂(x，y，z)， ξ₃＝f₃(x，y，z) とする。いま c₁，c₂，c₃ をそれぞれ定数とし，ξ₁＝c₁， ξ₂＝c₂， ξ₃＝c₃ とおけば，これらはすべて曲面を表わす。ここで c₁，c₂，c₃ の値を変化させれば，3種の曲面群が得られる。この3種の曲面群のそれぞれから，曲面を1つずつとったとき，それらが1点Pで交わり，また逆に，空間の任意の1点を通ってただ1通りの曲面の組 ξ₁＝c₁， ξ₂＝c₂， ξ₃＝c₃ があるとする。こう考えれば，空間の各点 (x，y，z) と実数の組 (ξ₁，ξ₂，ξ₃) とが一対一に対応することになる。すなわち，実数の組 (ξ₁，ξ₂，ξ₃) を，空間の点 (x，y，z) を定める座標とみなすことができる。この (ξ₁，ξ₂，ξ₃) を，3次元空間の点Pのまわりの曲線座標という。3つの関数 f₁(x，y，z) ，f₂(x，y，z) ，f₃(x，y，z) が，曲線座標 (ξ₁，ξ₂，ξ₃) を定めるための必要十分条件は，ヤコビアン (関数行列式) が0にならないことである。2次元や4次元以上の空間についても，同様である。

出典　ブリタニカ国際大百科事典小項目事典ブリタニカ国際大百科事典小項目事典について　情報

<<: Tamakawako - Gyokusenshi

>>: Curve Salvation - Kyokusenkyukoku