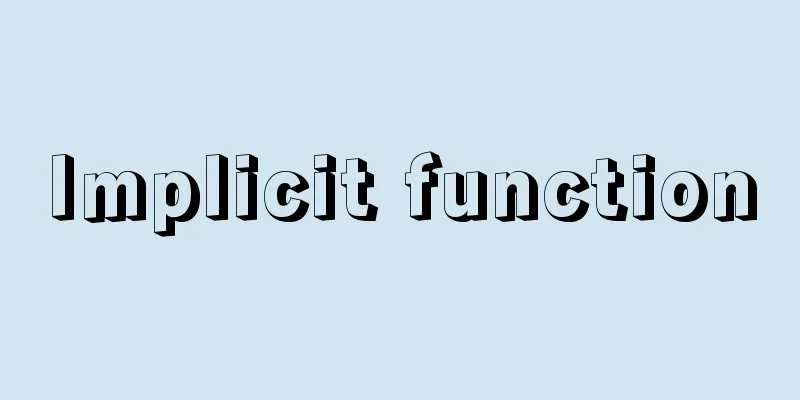
Implicit function

Japanese: 陰関数 - いんかんすう（英語表記）implicit function

Given an equation, for example x ² +y ² =1, if you define one value for x, you can find the corresponding value of y, and y is determined as a function of x. A function determined in this way is called an implicit function. In general, for two variables x and y, in an equation f(x,y)=0 where the functions of the two variables x and y are set to zero, if you can determine a single value of y that satisfies f(x,y)=0 when you define the value of x, then y becomes a function of x. This does not mean that the consideration is usually limited to a narrow range. In other words, for x ² +y ² =1, x must be in the range that satisfies -1≦x≦1, and even then, since x ² +y ² =1 generally gives two values of y, you must consider it near a certain point so that it is determined to be one.

For the above reasons, the following theorem is the basis for generally considering implicit functions (except when the function is given in a concrete form).

[Osamu Takenouchi]

Implicit Function Theorem

Let f(x,y) be a continuous function of two variables, x and y, and let its partial derivative with respect to y, f _y (x,y), exist and be continuous. Then,
f(x ₀ ,y ₀ )=0, f _y (x ₀ ,y ₀ )≠0
In the neighborhood of a point (x ₀ , y ₀ ) that satisfies, y can be expressed as a function of x. That is, if there is a continuous function g(x) defined in the neighborhood of x ₀ , then
g(x ₀ )=y ₀ , f(x,g(x))=0
holds true.

[Osamu Takenouchi]

[Reference] | Function

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

ある方程式、たとえばx²＋y²＝1が与えられると、xの値を一つ定めれば、これから対応するyの値が求められ、yはxの関数として定まる。このようにして定められる関数を陰関数という。一般に二つの変数x、yに対して、2変数x、yの関数をゼロと置いた方程式f(x,y)＝0において、xの値を決めたとき、yの値をf(x,y)＝0を満たすようにただ一つ決めることができれば、yはxの関数となる。これは通常狭い範囲に考察を限らないといえない。すなわちx²＋y²＝1では、xは－1≦x≦1を満たす範囲になければならず、またそのときも、x²＋y²＝1からは一般にyの値が二つ出てくるので、それが一つに定まるように、ある点の近くで考えることにしなければならない。

　前記のような事情から（関数が具体的な形で与えられた場合は別として）、一般に陰関数を考察するためには、次の定理が基礎とされる。

［竹之内脩］

陰関数定理

f(x,y)はx、y2変数の連続関数で、そのyに関する偏導関数f_y(x,y)が存在して連続であるとする。そのとき、
　　f(x₀,y₀)＝0,　f_y(x₀,y₀)≠0
を満足する点（x₀,y₀）の近傍において、yはxの関数として表すことができる。すなわち、x₀の近傍で定義された連続関数g(x)があって、
　　g(x₀)＝y₀，f(x,g(x))＝0
が成立する。