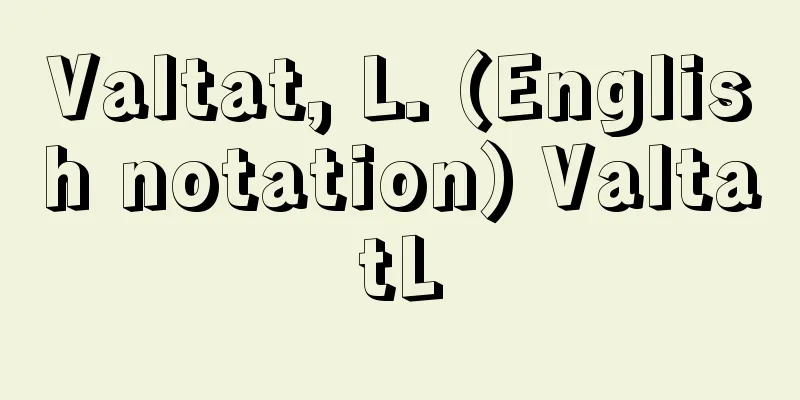
Operator - enzanshi (English spelling) operator

Japanese: 演算子 - えんざんし（英語表記）operator

Also called an operator, it is generally synonymous with mapping. It is often used in linear and function spaces. For example, when transforming a function f(x) into another function g(x), we write g(x)＝Tf(x).
where T is called an operator. Operators are often specified by certain rules of calculation. For example, the differential operator D, which is often used in calculus, means to differentiate a function f(x) to create f′(x), and is written as Df(x)＝f′(x).
D ² f(x)＝D［Df(x)］＝f″(x),……
It is.

Partial differentiation is an issue for a function f(x,y,z) defined at a point in space (x,y,z), and the following operators are often used as notation for vector analysis.

∇ is pronounced as nabla, ∇f is the gradient vector of the function f, and Δ is called the Laplacian (Laplace operator). Also, the vector value V(x,y,z)＝(f(x,y,z),g(x,y,z),h(x,y,z)) at the point (x,y,z)
For the function that corresponds to

If we define the operators div (divergence) and rot (rotation) by
divV = (∇, V),
rotV＝∇×V
The operator ∇ can be treated in the same way as a vector.

[Haruo Sunouchi]

Operators in Physics

Various concepts and methods of mathematics have been used in physics as much as possible. Operators are one of them, and operators are used in a wide variety of ways in physics today, especially because Heaviside, an electrical engineer, pioneered the use of operator theory after analyzing electrical circuits. Since most of physics is linear physics, most of the operators that appear in physics are linear operators and are treated as first-order maps in linear spaces or function spaces.

Operators play a particularly important role in the field of quantum mechanics. In quantum mechanics, the results obtained by measurement are probabilistic, so a distinction must be made between a "certain quantity" and its "measurement value." For this reason, we consider a function that represents a state (a state function) and an operator that represents a "certain quantity" that acts on it. This is particularly important when the result of applying an operator is a fixed numerical value, and in such cases the operator represents a "physical quantity." In other words, the result of a measurement is always uniquely determined. This provides the basis for measurement in quantum mechanics. The state at this time is called an eigenstate of that physical quantity, and is an eigenfunction of the operator. A general state is then expressed as a superposition of these eigenstates. In this way, in quantum mechanics, all physical quantities are expressed by operators, which are linear due to the principle of superposition, and have self-conjugacy (Hermitianity) due to the requirement that all observed values be real numbers.

Since operators generally cannot be switched in the order in which they are applied, two physical quantities represented by them will not give the same results when they are applied to a state in the switched order. In other words, the same measured values will not be obtained. This is the content of the uncertainty principle, and only in the special case in which two operators are interchangeable can the two physical quantities be observed simultaneously.

[Jun Fujimura]

[Reference] | Calculus method | Mapping | Vector analysis

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

作用素ともいい、一般には写像とほぼ同義の内容をもつ。とくに線形空間や関数空間で用いられることが多く、たとえば関数f(x)を他の関数g(x)に変換するとき、それを
　　g(x)＝Tf(x)
のように書いて、Tを演算子という。演算子はしばしば一定の計算規則で指定されている。たとえば、演算子法でよく用いられる微分演算子Dは関数f(x)を微分してf′(x)をつくることを意味し
　　Df(x)＝f′(x),
　　　D²f(x)＝D［Df(x)］＝f″(x),……
である。

　空間の点（x,y,z）で定義された関数f(x,y,z)では偏微分が問題になるが、ベクトル解析の記法として、次のような演算子がよく用いられる。

∇はナブラと読み、∇fは関数fの勾配(こうばい)ベクトル、Δはラプラシアン（ラプラス演算子）という。また、点（x,y,z）にベクトルの値
　　V(x,y,z)＝(f(x,y,z),g(x,y,z),h(x,y,z))
を対応させる関数に対し、

によって、演算子div（発散）とrot（回転）を定義すると、
　　divV＝(∇,V),
　　rotV＝∇×V
などの関係があり、演算子∇をベクトルと同様に取り扱うことができる。

［洲之内治男］

物理学における演算子

数学のさまざまな概念や手法は可能な限り物理学のなかで用いられてきた。演算子もその一つであるが、とくに電気工学者であったヘビサイドが電気回路の解析をきっかけに演算子法を開拓したという事情もあり、今日物理学のなかで演算子は非常に多彩に用いられている。物理学のほとんどの部分が線形物理学であるから、物理学に表れる演算子は多く線形演算子であり、線形空間あるいは関数空間の一次写像として扱われる。

　演算子がとくに重要な役割をもつのは量子力学の分野である。量子力学では、測定により得られる結果は確率的なので、「ある量」とその「測定値」とは区別されなければならない。このため状態を表す関数（状態関数）と、それに作用する「ある量」を表す演算子を考える。演算子を作用させた結果が定まった数値を与える場合はとくに重要で、このような場合、演算子は「物理量」を表している。すなわち、一つの測定の結果がかならず一義的に定まっている。これが量子力学における測定の基礎を与える。このときの状態はその物理量の固有状態といわれ、演算子の固有関数である。そして一般の状態はこれら固有状態の重ね合わせで表される。このように、量子力学ではすべての物理量は演算子で表され、その演算子は重ね合わせの原理により線形であり、また観測値はすべて実数であるという要請から自己共役(きょうやく)性（エルミート性）をもつ。

　演算子は一般に作用させる順序を交換できないから、それで表される二つの物理量は、順序を入れ替えて状態に作用させたとき、等しい結果を与えない。すなわち同じ測定値は得られない。このことが不確定性原理の内容であり、とくに二つの演算子が交換可能な特別の場合にだけ、その二つの物理量は同時に観測可能となる。

［藤村　淳］

[参照項目] | 演算子法 | 写像 | ベクトル解析

出典　小学館　日本大百科全書(ニッポニカ)日本大百科全書(ニッポニカ)について　情報 | 凡例

<<: 《Operative method》

>>: Cocaine hydrochloride