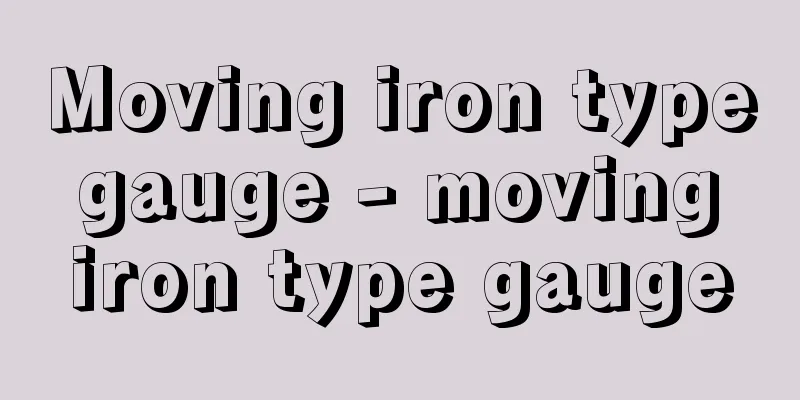Algebraic equations

Japanese: 代数方程式 - だいすうほうていしき

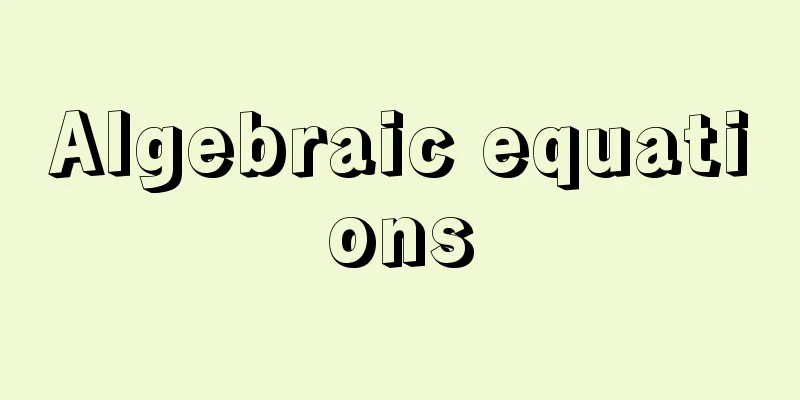
An equation with unknowns x, y, z, etc. is f(x,y,z,……)=0
When written in this form, if the expression on the left hand side is an algebraic expression (rational or irrational), it is called an algebraic equation.

[Tsuneo Adachi]

Binomial Equations

An equation of the form X ^m -a=0 is called a binomial equation. One of its solutions is expressed as ^m or a ^1/m . When a is a positive real number, ^m is usually a positive real number.

[Tsuneo Adachi]

Cubic Equation

Cubic equation a ₀ X ³ +a ₁ X ² +a ₂ X+a ₃ =0
(a ₀ ≠ 0)
To solve this, first use the variable transformation x=ya ₁ /3a ₀ to get X ³ +3pX+q=0…………〔1〕
It is transformed into the following form:

Then, the solution to (1) is α ^1/3 +β ^1/3 ,
ωα ^1/3 +ω ² β ^1/3 ,
^ω2α1 ^/3 +ωβ1 ^/3
Here, ω is (-1 +)/2. This formula is called Cardano's formula. Quartic equations can be solved by reducing them to cubic equations. The formula for this solution is called Ferrari's formula.

[Tsuneo Adachi]

Fundamental Theorem of Algebra

An n-th degree algebraic equation over the field of complex numbers (complex numbers include the case where they are real numbers. The same applies below) is f(x)=a ₀ X ⁿ +a ₁ X ^n-1 +……
+a _{n -1} X +a _n = 0 ... (2)
(a ₀ (≠0), a ₁ ,……
, a _n is a complex number)
The polynomial on the left side of [2] is always f(x)=a ₀ (X-α ₁ )……(X-α _n ).
(α ₁ ,……,α _n are complex numbers)
and a product of linear expressions. In other words, an n-th degree algebraic equation has n solutions if multiplicities are also taken into account. This is a theorem proven by Gauss, and is called the Fundamental Theorem of Classical Algebra. This property, that is, the property that an algebraic equation with complex coefficients always has a complex solution, is expressed as saying that the field of complex numbers is algebraically closed. For example, even an algebraic equation with rational coefficients does not necessarily have a rational solution, so the field of rational numbers is not algebraically closed. In this sense, we can see that the Fundamental Theorem of Classical Algebra is a theorem that shows the completeness of the field of complex numbers.

[Tsuneo Adachi]

Equations of degree 5 or higher

Algebraic equations up to degree four can be solved by using power roots, that is, by repeatedly solving binomial equations. This property means that algebraic equations of degree four or less can be solved algebraically.

In the early 19th century, Abel proved that algebraic equations of degree five or higher could not in general be solved algebraically. Galois then introduced the concept of groups and sought necessary and sufficient conditions for algebraic equations to be solvable algebraically. This was the beginning of group theory.

[Tsuneo Adachi]

Multivariate Algebraic Equations

When there is more than one unknown, an algebraic equation can have a meaning in plane figures, space figures, or even higher dimensional figures. For example, x ² +y ² -1=0
represents a circle. Algebraic geometry is the study of algebraic equations from a geometrical standpoint, and this field has made remarkable progress in recent years.

[Tsuneo Adachi]

[Reference item] | Group | Cubic equation

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

未知数x、y、z、……を含む方程式を
　　f(x,y,z,……)=0
の形で書くとき、この左辺の式が代数式（有理式あるいは無理式）である方程式を代数方程式という。

［足立恒雄］

二項方程式

X^m-a=0の形の方程式を二項方程式という。その解の一つを^mまたはa^1/mで表す。aが正実数のときには^mは正実数とするのが普通である。

［足立恒雄］

三次方程式

三次方程式
　a₀X³+a₁X²+a₂X+a₃=0
　(a₀≠0)
を解くには、まず変数変換x=y-a₁/3a₀により
　X³+3pX+q=0…………〔1〕
の形に変形する。次に

と置くと、〔1〕の解は
　　α^1/3+β^1/3,
　　ωα^1/3+ω²β^1/3,
　　ω²α^1/3+ωβ^1/3
で与えられる。ここにωは（-1+）/2である。この公式をカルダーノの公式という。四次方程式は三次方程式に還元して解かれる。その解の公式をフェラリの公式という。

［足立恒雄］

代数学の基本定理

複素数体上のn次代数方程式（複素数は実数である場合を含めていう。以下同様）とは
　f(x)=a₀Xⁿ+a₁X^n-1+……
　　　+a_n-1X+a_n=0……〔2〕
　　　(a₀(≠0),a₁,……
　　　,a_nは複素数)
という形の方程式である。〔2〕の左辺の多項式はかならず
　f(x)=a₀(X-α₁)……(X-α_n)
　(α₁,……,α_nは複素数)
と一次式の積に分解できる。すなわちn次代数方程式は重複度も考慮すればn個の解を有する。これはガウスによって証明された定理で、古典代数学の基本定理とよばれる。この性質、すなわち、複素係数の代数方程式はかならず複素数解をもつという性質を、複素数体は代数的に閉じていると言い表す。たとえば有理係数の代数方程式でも、かならずしも有理数解をもつとは限らないから、有理数体は代数的に閉じていない。こういう意味から、古典代数学の基本定理は複素数体の完全性を表す一つの定理であることがわかる。