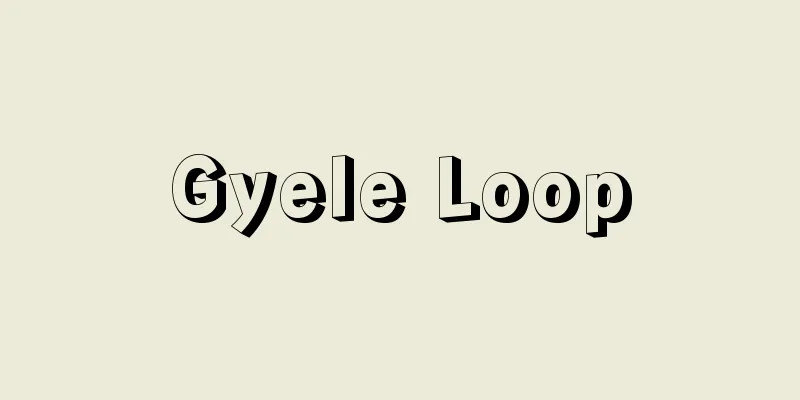Inflection point

Japanese: 変曲点 - へんきょくてん

It is the point where the curve changes its concave/convex state. A curve y = f ( x ) on the xy _{plane is convex downward (or concave upward) if the point on the curve between them is always below the line segment P1P2 when a ≦ x ≦ b holds two arbitrary points P1(x1} , f ₍ _x1 ) ) _, P2 ( x2 _, _f ( x2 )) and is always convex upward (or concave downward) if it is always above _the line segment _P1P2 ( Figure (1)). In this case, f ( x ) is continuous except for x = a , x = b , and has a differential coefficient on one side at each point. Also, if f ″( x ) exists, then y = f ( x ) is convex downward in the interval where f ″( x ) ≧ 0 holds, and is convex upward in the interval where f ″( x ) ≦ 0 holds.

If a curve y = f ( x ) has a point x = c that is convex downward for x ≦ c and convex upward for x ≧ c (or vice versa), then the point _P0 ( c , f ( c )) is an inflection point of the curve ( Figure (2)). If f "( x ) exists near x = c and f "( x ) changes sign before and after x = c , then _P0 ( c , f ( c )) is an inflection point of the curve. If a tangent is drawn to the curve at the inflection point, the curve is on the opposite side of the tangent before and after the inflection point. Also, the sign of the curvature is reversed before and after the inflection point (the curvature is 0 at the inflection point).

[Osamu Takenouchi]

Inflection point explanation diagram [figure]
©Shogakukan ">

Inflection point explanation diagram [figure]

Source: Shogakukan Encyclopedia Nipponica About Encyclopedia Nipponica Information | Legend

Japanese:

曲線が凹凸の状態を変える点をいう。xy平面上の曲線y=f(x)は、a≦x≦bにおいて、その点の任意の2点P₁(x₁,f(x₁)),P₂(x₂,f(x₂))をとるとき、その間にある曲線上の点が、つねに線分P₁P₂より下側にあるならば下に凸（あるいは上に凹）、つねに上側にあるならば上に凸（あるいは下に凹）という（図の(1)）。このとき、f(x)は、x=a,x=bを除いては連続、かつ各点において、片側の微分係数をもつ。また、f″(x)が存在すれば、y=f(x)は、f″(x)≧0が成立する区間で下に凸、f″(x)≦0が成立する区間で上に凸である。

　曲線y=f(x)において、ある点x=cについて、その近くで、x≦cに対して下に凸、x≧cに対して上に凸（あるいはその反対）であるならば、点P₀(c,f(c))はこの曲線の変曲点である（図の(2)）。もし、x=cの近傍において、f″(x)が存在し、f″(x)がx=cの前後で符号を変ずるならば、P₀(c,f(c))はこの曲線の変曲点である。変曲点で曲線に接線を引くと、変曲点の前後において、曲線はこの接線の反対の側にある。また、変曲点の前後において、曲率の符号は逆になる（変曲点では曲率は0）。